Making Angles – Elementary Math

Purpose

To explore different types of angles including acute, right and obtuse

Materials

Pencils or Cuisanaire© rods

Overview

To help students practice the terms acute, right, and obtuse angles, ask them to make various types of angles using two pencils or Cuisenaire© rods and describe them in words. Some angles they might create are:

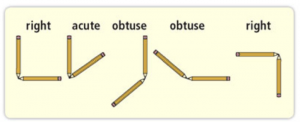
angles

When there is a disagreement about whether a particular angle fits the classification you gave, allow a student to compare the angle to the corner of a pieces of paper (a right angle). See how many 90 degree right angles they can make with pencils or rods facing different directions. Then have children make acute and obtuse angles. To extend the activity, call out a angle measurement and have students show the kind of angle (not exact measurement) needed to equal 180 degrees. For example, if I say 45 degrees (acute angle), students create an obtuse angle because this is the type of angle needed to measure 180 degrees.

About the sequence

Part 1 asks students to use two pencils or Cuisanaire© Rods to make right angles. Part 2 asks students to use the pencils or rods to make acute and obtuse angles. The extension is based on students’ understanding that a straight line = 180 degrees.

Part 1

Let’s use our pencils/rods to create right angles. Once you have 1 right angle, can you use your pencils to create another right angle facing a different direction? Remember you can use the corner of your paper to visualize the exact measurement of a right angle.

Examples:

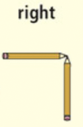
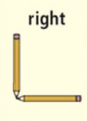
right angle

While children are enjoying their building of mastery, feel free to repeat. When children are eager for more, try Part 2.

Part 2

Now, let’s use our pencils or rods to create some acute angles (less than 90 degrees) as well as some obtuse angles (more than 90 degrees). Once you’ve created one of each type of angle, see if you can make other examples of each angle with your pencils laid out differently.

Examples:

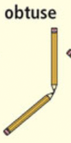
obtuse angle

As always, when children seem excited for a new challenge, move on.

Extension

I’ll name a measurement, then you use your pencils to model whether a right, acute or obtuse angle is needed for the sum of the two angles to equal a total of 180 degrees.

Examples:

50 degrees (needs an obtuse angle)
120 degrees (needs an acute angle)
93 degrees (needs an acute angle)
90 degrees (needs a right angle)