Language, mathematics, and habits of mind

E. Paul Goldenberg, June Mark, and Al Cuoco Education Development Center, Inc. (EDC)

See version that appeared in NCTM’s Teaching Children Mathematics

When should algebra be taught?

Asking “When should algebra be taught?” is like asking “Is technology harmful or helpful?” There are lots of technologies and lots of uses of them. Some are harmful; some are helpful. Refining the question—asking about a particular use of a particular technology for a particular purpose in particular contexts and at particular stages in one’s learning—makes the question researchable and potentially answerable. Similarly, there are many “algebras”—algebra the course, algebra the discipline, algebraic ideas, algebraic language, early algebra, “patterns, functions, and algebra”—and many different takes on the learning and teaching of each of these. Treating algebra as an indivisible whole obscures the options. It’s more useful to ask what ideas, logic, techniques, and habits of mind algebra entails, and then, about each of these, ask when and to what extent that one item can be learned with intellectual integrity and how a coherent whole can be woven out of these learnings. The answers we get are that some of these ideas do have to wait for eighth or ninth grade, but that others—even including aspects of algebraic language—are already there, early in the primary grades. Moreover, children who get to apply, refine, and strengthen those ideas and skills as they emerge gain the advantage.

Any credible claim about habits of mind must surely accord with features of mind: children’s cognitive development. For a charmingly written scientific account of the ways that babies and young children think, read The Scientist in the Crib.[2] The habits of mind approach to curriculum that we first described well over a decade ago[3],[4] and have continued to refine[5],[6],[7],[8] does accord well with children’s thinking and became a central design principle behind Think Math![9], the newest NSF-supported elementary curriculum, developed at EDC.

Recognizing, enhancing, and building on developmentally natural habits of mind lets us dissect algebra and sort the resulting bits and pieces in a developmentally natural way, while preserving the content, concepts, and skills that schools (and states, parents, workplaces, and colleges) expect. The fact that it is possible to organize algebraic ideas, logic, and techniques around the development of mind makes clear that we are truly talking about thinking—habits of mind—rather than “features of mathematics” or “idiosyncrasies of mathematicians.” This article describes two of these natural habits of mind.

Two algebraic ideas that precede arithmetic

The common wisdom is arithmetic first, algebra later. The truth is not so simple. Some algebraic ideas—ideas about the properties of binary operations apart from the numbers these operations may “combine”—develop naturally before children learn arithmetic.

In fact, they must develop before arithmetic can make sense! For example, for many 4-year-olds, even those who appear to count well, seven objects spread out like this  feel like “more” than the same objects bunched together . (Though “conservation” remains the familiar name for this stage in children’s logic—so we’ll still use it—child logic is more nuanced than was previously thought. It’s known, for example, that for small enough numbers of objects, babies at eleven months have not only stability of number but essentially addition as well.[10] So-called non-conservers aren’t “enslaved by their senses” but haven’t yet privileged the analytic act of counting over other ways of making social and mathematical sense of the world.) For children whose logic still works that way, the claim that + is the same amount as can hardly make sense. Faced with the requirement to assert that 5 + 2 = 7, “non-conservers” have only two options. Some divorce the assertion from their current “common sense”—after all, they “know” that the two quantities are not the same—and learn “5 + 2 = 7” as an arbitrary but learnable fact, the same way they learn the names of their classmates. For them, math is memory. Others find it hard to accept what their logic tells them is “not true” and, instead, just feel like they “don’t get it.”

An important property of addition before addition, itself

What will later be formalized as the commutative and associative laws of addition begins as an intuitive sense of stability/invariance of quantity under rearrangement. Piaget[11] called it conservation of number; Wirtz, et al.[12] and Sawyer[13] called it the “any order any grouping property.” Prior to conservation, while arrangement trumps number, 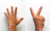may not have a fixed number associated with it. Later, the new conserver may not yet know how many fingers are without counting, but will be sure that the number, whatever it is, stays put if the hands are moved like this or even like this, .

That algebraic idea, a property of aggregation, must exist before the arithmetic fact—knowing what number 2 + 5 is—can make sense. In a similar way, if a bunch of coins are hidden and we ask “how much money is there?” children for whom the question makes any sense will be absolutely certain that there is an answer, and that only one answer is correct. They may be uncertain about methods of counting, and may think that some methods might give incorrect answers. The complexities of communication may even make it seem that they believe that the amount, itself, could vary depending on what method they use as they count but, in all likelihood, other means of questioning would suggest that they’re sure that the amount is stable. In fact, if they do believe the amount can vary, they’re not cognitively ready for the question of what “the amount” is. There is no “the amount” if it can vary. Some six year olds, but not many, do not yet conserve number; by seven, nearly all do.

Having confidence that and  represent the same quantity is not the same as knowing the commutative property of addition. The commutative property is not about the arrangement of physical objects in space, but about the behavior of a particular element (here, the + sign) in a formal syntactic system of written symbols. In some contexts, children can make perfect sense out of written symbols—even significant parts of algebraic notation—but most young children cannot make sense of formal operations on a string of symbols. So, at this stage, commutativity remains largely an intuitively obvious idea about the “physics of mathematics”: the nature of aggregation, not the nature of symbols. Even so, we, as educators, can support the young child’s logic better if we recognize that it is already relying on the underlying ideas that formal mathematics will later codify. The fact that children see that the principle applies regardless of the numbers means that it captures the essential algebraic aspect of the structure of addition that commutativity is about.

Logical precursors of the distributive property of multiplication over addition

Pick a number. Multiply it by 5; also multiply it (your original number) by 2; now add those results. You get the same answer you’d get if you multiplied your original number by 7. The distributive property, a general statement of that fact, is possibly the most central idea in elementary arithmetic, key to understanding the algorithms, at the core of fluent mental calculations (e.g., 102 × 27 can be computed in two parts, as 100 × 27 + 2 × 27), and the logical basis for many “rules” of algebra that might otherwise seem arbitrary.

This property relates multiplication and addition, but children “know it” long before they even meet multiplication! It’s in the language (and logic) they use when they say that 5 (fingers, pennies, or 27s) plus 2 (fingers, pennies, 27s) make 7 (fingers, pennies, 27s). These dialogues with 6-year-olds, late in their kindergarten year, give a sense of what their logic does and does not handle. What distinguishes the questions the children get “right” from those they get “wrong”? What logic might explain the particular wrong answers they get?

T What’s a really big number?
Ne (girl): A million!
T: Suppose I said “How much is a thousand plus a thousand?” What would you say?
Ne: I have no idea! (big smile)
T: And suppose I said “How much is two thousand plus three thousand?”
Ne: (thinks, then confidently) Five thousand!

T: Suppose I said “How much is a hundred plus a hundred?” What would you say?
Gi (girl): A hundred.
T: What about “Two hundred plus three hundred”?
Gi: Five hundred.
T: (playfully) And what if I said “how much is a thousand plus a thousand?” …
Gi: A million!

T: Suppose I said “How much is a hundred plus a hundred?” What would you say?
De (boy): De may hear “a hundred” as one word, so confidently says: Two ahundred.
T: And suppose I said “How much is two hundred plus three hundred?”
De: Five hundred.

T: Suppose I said “How much is a thousand plus a thousand?” What would you say?
Co (boy): A thousand two. (Co might have meant “A thousand, too.” We don’t know.)
T: And suppose I said “How much is two thousand plus three thousand?”
Co: Two three a thousand. (Co clearly isn’t yet adding naturally.)

As soon as children are comfortable with the idea (and language and knowledge) to answer “what’s three sheep plus two sheep?” perhaps late in K or early in first grade, they’ll happily apply that to give the “correct” answer to the spoken question “what’s three eighths plus two eighths?” or “what’s three hundred plus two hundred?” The answer is “correct,” but what they have in mind may well be quite different from what we have in mind when we give the same answer. We can see how different their ideas are when we ask a slightly different question: “what’s a hundred plus a hundred” (with no audible “small” numbers like “two” or “three”). To this question, young six-year-olds may well repeat “a hundred” or say something like “a million.” If, instead, we ask “what’s an eighth plus an eighth,” little ones may just give a puzzled stare and not answer at all; or, if their arithmetic is strong enough, they might possibly count and answer “sixteen” (or, sometimes “nine”).

How can we explain such different responses to questions that adults see as so similar? Again, the answer rests more in language and general cognition than mathematics. Kindergarteners typically have hundred and half as vocabulary items. For most little ones, these terms don’t represent precise or fixed amounts, just as “a zillion” is not a specific fixed amount to us, but the children do know that “half” means only part. Most even know that halves should be equal— no fair if yours is bigger!—though they might not know that they must be equal or that there are only two of them. And they almost certainly don’t know that half is a number. Likewise, they know that “a hundred” is big, though they are unlikely to know how big. The question “what’s a hundred plus a hundred” is, therefore, more or less, “what is a big amount plus another big amount?” The natural response is “a big amount” (“a hundred”) or a very big amount (“a million”), not “two big amounts” (“two hundred”). But when fixed specific quantities are available, children use them. The question “what’s two hundred plus three hundred” is linguistically and cognitively like “what’s two sheep plus three sheep”—it draws attention to 2 + 3, not to the nature of a sheep or a hundred. Children for whom 2 + 3 makes sense answer correctly. Of course, children for whom 2 + 3 does not yet make sense try to find some other way of making sense of the task, but their answers don’t reflect addition. (The different response to “what’s an eighth plus an eighth”—the puzzled look—is because an eighth not even part of the child’s vocabulary, and thus, with no meaning, gives the child less of a context for responding. Anna Sfard[14] suggests that a child might well treat “hundred” as a number, rather than a sheep, and still treat “three hundred” not as a number, but as an expression composed of two number words. If so, our kindergarteners seem to treat these numbers differently, one as a counter, the other as a unit or object, which might be consistent with Sfard.)

Why these errors are made, and why “hundred” and “eighth” lead to different errors, is a diversion. The point is that when no audible small numbers like “two” or “three” are given, little children tend to give wrong answers. But when we say how many eighths or hundreds, and the numbers are not too large, even kindergarteners tend to answer correctly, more first graders do, and we can absolutely count on it in second grade. Whatever an eighth or a hundred is, the children are sure that three of them plus two of them is five of them! This does not constitute “knowing the distributive property,” but it does tell us that the children already have the underlying idea that the distributive property will later encode formally.

If we use sevens (a fully understood fixed quantity) in place of hundred (which may still be a nonspecific “zillion” for young children), children still know that three of them plus two of them makes five of them, but that’s of little use if “three sevens” does not (yet) have meaning. Once a child does have meaning for “three sevens” and that meaning is a specific number (even if the child doesn’t yet remember which number), the child’s long-standing logic/intuition/linguistic knowledge that “three sevens plus two sevens is five sevens” becomes arithmetically usable.

The meaning of “three sevens” might be given in several ways: as an image , or a sum, 7 + 7 + 7, or a product 3 × 7, or in other ways. Each way has something threeish and something sevenish about it. Because 7 + 7 + 7 and 3 × 7 are both language, such expressions are best introduced as (mathematical) descriptions of a situation—for example, the array image—that communicates partly without analyzing the language formally. The image, of course, requires some analysis, too—visual rather than linguistic—to see the three sevens. To connect “three sevens” with 21, the “normal” name for that number, we must agree that what makes Image:1sevens.png “seven” is its seven squares. Then is 21 because of its 21 squares, but it is also a picture of three sevens: a multiplication fact. Similarly, if is “seven,” then is two sevens. The picture shows that three sevens and two sevens make five sevens.

In spoken form, “three sevens plus two sevens make five sevens” is familiar. The pictures support the semantics of the situation, helping to establish the role of sevens and preserve its numerical meaning rather than letting it degenerate into a non-numeric object, like sheep. But the classical written form—(3 × 7) + (2 × 7) = 5 × 7—is quite another story.

Spoken symbols vs. written symbols

Knowing that the finger collections and  can be described by the same number does not guarantee that a child will know that the print statements 5 + 2 and 2 + 5 refer to the same number. The written language of mathematics presents challenges that can be finessed by spoken language and by appropriate visual presentations. Perhaps the most glaring example is the canonical wrong fourth-grade response to . No first grader would ever say “five sixteenths.” It’s uninformative—in fact, misleading—to “explain” such errors simply by claiming that these expressions are “too abstract” or that children “can’t handle symbols.” Spoken words are symbols, too, and words like the—which young children use flawlessly—are about as abstract as one can get. It’s worth understanding the difference between = and 5 + 2 = 2 + 5 to see why the challenge of print for children may not be a mathematical challenge.

Humans have evolved to be quite flexible about visual order and orientation, but in the life of any individual human, it takes some learning. Infants who have come to recognize a bottle when it is handed to them in the proper orientation Image:BabyBottleNippleDown.png do not, at first, reach for it when it is handed to them in some unfamiliar orientation (e.g., with the nipple visible, but facing away like this Image:BabyBottleNippleAway.png). But very soon they do learn to recognize objects regardless of their orientation. When you consider the visual processing required, this is quite an impressive accomplishment. Even if the bottle is presented in the same orientation but at different distances, very different images are projected onto the retina. The distortion of parts relative to each other can be extreme, and yet the baby recognizes all of these projections—most of them never seen before—as the same object.[Figure 1: In this photo, the distance from the tip of the nipple to the bottle is the same as the length of the entire rest of the bottle. Measure to see for yourself!]Though this complex neural computation needs data (learning) to tune it up, the ability, itself, is wired in. This evolutionary gift is essential for survival. Otherwise, we’d have been meals for tigers we didn’t recognize because they didn’t happen to be facing exactly the same way as first we saw them! For our ancestors, it was necessary to “see” the same object despite different retinal images, as long as those images could be made “the same” under rotation, reflection, dilation, or certain projective transformations, and so our brains are adept at them. (The spatial tests that some people find quite difficult are a very different sort of thing. The “look-alike” objects on these tests require an analysis that goes beyond what was evolutionarily useful. Our ancestors didn’t care if the tiger was left-handed!)But those ancestors didn’t read. The letters d, b, q, and p are the same shape and differ only by rotation or reflection. To read, children must learn to see them as different objects, not as the same object in different orientations. Young children’s letter reversals are not neurological failures at all—seeing that way is one of evolution’s gifts—but, just for this one purpose of decoding print, children must unlearn a principle that applies to nearly everything else they will encounter during their entire life. They must treat print as an exception to the usual rules of seeing.Moreover, was and saw — each just three print-squiggles arranged in a different order—must not be recognized as “the same.” Alas, then come 2+5 and 5+2, two perfectly good examples of print-squiggles that are to be treated as “the same.” (As always, the truth is not so simple. On a number line, numbers represent addresses — the names of specific points/locations along the line — and also distances between addresses. The child who “enacts” 2+5, perhaps by jumping along a large number line on the floor would enact 5+2 differently.) It is therefore not surprising that the notation, in some contexts, can cause confusions, but this is an issue of notation, not of concept. Print is just plain different!Similarly, the picture lets children see what written descriptions like (3 × 7) + (2 × 7) = (3 + 2) × 7 or (3 × 7) + (2 × 7) = 5 × 7 typically leave opaque, unless they are written as an abbreviated version of language the children themselves are using to describe the picture. But the difficulty is with the notation—a difficulty with the manner in which the underlying mathematical idea is being communicated—not a lack of the idea itself. In fact, the way that teachers of kindergarten and early first grade teach writing could help them teach this symbolic language, too: children tell stories, and the teacher encodes their language in writing. Here, children might describe how a three-by-seven array can be put with a two-by-seven array to make a five-by-seven array, and the teacher can be writing (3 × 7) + (2 × 7) = (5 × 7) as the children speak. Before that can happen, children need to have the idea that we can name the arrays, and that one useful name for is (3 × 7). Imagine that array to be on a card we hold in our hands. That card can be held in any position at all—vertically, slantwise, horizontally—and is still the same card. It makes sense to give it the same name no matter which way we hold it. We could also have called it (7 × 3), or even 21 (or a zillion other things, like “half of 6 × 7” if we had a 6 × 7 array that we had already named). So (3 × 7) = (7 × 3) =…The visual idea and the symbols that describe what the children see are not yet fully generic—not yet a property of + and × that can be used in syntactic manipulations of strings of symbols to generate (a × c) + (b × c) from (a + b) × c or vice versa. In fact, there are so many parts to keep track of that doing so is not trivial. Getting good enough to recognize and use this valuable property, even with arrays as a particularly powerful representation, takes time and practice. But the underlying idea is there very early, as part of the child’s cognitive structure, as soon as the child can meaningfully make statements like “two sheep plus three sheep are five sheep.” Again, the underlying idea must be there before any practice of it can make sense.Written symbols often present major challenges that the spoken symbols do not. Possibly because of print’s special status, the logic that children apply when information is presented in spoken symbols may not be applied when the same information is presented in print. The canonical error with fractions is a perfect example: The spoken question “what’s three eighths plus two eighths” focuses attention on “three plus two” and tends to evoke the correct reasoning and get the correct answer; by contrast, the written question 3/8 + 2/8 = ?  doesn’t focus attention only on the top numbers. Children for whom the meaning is not already strongly established tend to interpret the plus sign as “add everything in sight.”

In fact, mathematical reading and writing are quite different from prose reading and writing. For prose, we proceed in a line, strictly left to right. Even top-to-bottom movement just accommodates the limited width of a page; it gives no information that would not have been present if the writing were strung out in one dimension—a line—on a very wide scroll of paper. (The real story is, of course, more complex. Strict left to right reading applies only at the very earliest stages, if at all. A fluent reader, largely without conscious awareness, takes in much more of the sentence than a strictly left-to-right approach would give.) By contrast, bar graphs, coordinate graphs, histograms, charts and tables, and the like are two-dimensional records. One must attend to horizontal and vertical position in order to interpret the information they contain. Even symbolic expressions can require attention to vertical as well as horizontal position: 32 is not the same as 32. Moreover, mathematical writing that is just horizontal are not to be read strictly left to right: 2 × (3 + 5), 7 + 6 ÷ 2, and 7 + ___ = 5 + 4 all require attention to the right side before attention to the left. In fact, 7 + 6 ÷ 2 requires both left-to-right and right-to-left analysis: 6 ÷ 2 must be evaluated left-to-right (because 2 ÷ 6 is different), and yet the convention about order of operations dictates that the 6 ÷ 2 part must be evaluated before the addition that is specified by “7 + .”[Figure 2: Bar graphs, among the earliest graphs children make, require attention to two dimensions: which bar (horizontal position) and the bar’s height.]

Algebra as a language for expressing what we know

Algebraic notation is used in two distinct ways: for describing what we know, and for deriving what we don’t know. In the first use, algebra is a language for describing the structure of a computation, a numerical pattern we’ve observed, a relationship among varying quantities, and so on. Young children are phenomenal language learners! Exercises like the one in Figure 3, but without the leftmost column, are familiar enough in many curricula. Children look for a pattern in the inputs and outputs, figure out a rule, and complete the table. Think Math! often adds a “pattern indicator” (the first column) to problems of this kind. When Michelle, a second grader in a Think Math! classroom finished filling out this table before I had finished handing out copies to all the children, I asked her how she had done it so fast. She said “Well, I saw it was take-away 8 because I looked at the 28 and 20, and then I saw that 10 and 2 was take-away 8 again, and then I saw 8 and 0.”

Figure 3: A “pattern indicator” gains meaning from context when it accompanies a “find-a-rule” exercise.

And then she grinned as if I had left the “clue” by accident, pointed to the left column and added “Besides, it says that right here!” How did Michelle know? Though the algebraic language was there, nobody ever discussed “variables” or “letters standing for numbers” or even mentioned that column. Had Michelle seen just the table in Figure 4, with no examples to infer from, she most likely would not have felt the symbols “said” anything. But after she discovered the pattern, the symbols looked “close enough” to mean the same thing, and so she then assigned them that meaning.

Figure 4: A “pattern indicator” without a pattern from which to infer its meaning would just be more to learn.

In other words, she did what little children excel at: she learned language (in this case “n – 8”) from context. If algebraic language is part of the environment, used where context gives it meaning, children can apply their natural—and extraordinary—language-learning prowess to it, and learn to use it descriptively. Just as children learning their native language understand, at first, more than they can say, Michelle could not immediately produce such descriptive language, but she and others try these interesting ways of writing down what they know and, over time, become good at it.

Fourth graders learn a number trick: Think of a number; add 3; double that; subtract 4; cut that in half; subtract your original number; aha, your result is 1! They love it and want to do it to their parents and friends. They also want to know how it works, so we add pictures. When we say Think of a number, we picture a bag with that number of grapes in it:  . For add 3, we picture and double that becomes . This act of doubling, which most fourth graders find quite natural and “obvious,” is, again, the distributive property in action. While the expression 2(b + 3) does not make obvious what the result is, children do readily learn to describe the picture as “two bags plus 6” and abbreviate that description as 2b + 6. We don’t talk about “variables” or “letters standing for numbers”; we simply describe what we know, and write it down as simply as we can. (See a detailed description of the activity with children at Algebraic thinking and see Sawyer (1964)[13] for the original source of this idea.) June Mark, et al., (2009)[8] describe yet another way in which Think Math! gives students this algebra-as-descripith children at Algebraic thinking and see Sawyer (1964)[13] for the original source of this idea.) June Mark, et al., (2009)[8] describe yet another way in which Think Math! gives students this algebra-as-description-of-what-you-know experience.tion-of-what-you-know experience.ith children at Algebraic thinking and see Sawyer (1964)[13] for the original source of this idea.) June Mark, et al., (2009)[8] describe yet another way in which Think Math! gives students this algebra-as-description-of-what-you-know experience.

So why don’t we teach algebra-the-course in grade 4?

Because that other use of algebra—deriving what we don’t know—is a formal syntactic operation on a set of symbols, and children are (generally) not able to divorce symbols from meanings before roughly age 12. This is not because they cannot handle “symbolic” or “abstract” things—words are symbols; pictures are symbols; little children can be symbolic and abstract from babyhood—but because the use of the symbols is different. Formal operations on strings of algebraic symbols—rearranging them, apart from their semantics, to create other strings of symbols that “solve” a problem—are, well, formal operations, and children are not, by and large, formal operational before 11, and not reliably so before about 13, whence the common need to wait until that age for “algebra.” But only that part of algebra that requires deduction by formal rules needs to wait that long. The part of algebra that is expressive of what we already know—that is, essentially, a shorthand for semantic content clearly tied to a context we already understand—that part can be learned earlier. It is just language to express oneself, and children are excellent language learners. They don’t learn language from explanations or formal lessons; they learn it from use in context. And, if is it learned all along, as it becomes developmentally possible, then, when the child is in late middle school, the transition to the new use of that language for deductive purposes could, presumably, be much easier, much more accessible for all children, much less of a brick wall of a million seemingly new things to learn all at once.

What does this tell us about elementary school teaching and learning?

Taking advantage of children’s natural algebraic ideas and honing them is a focus on habits of mind, rather than on rules that can otherwise seem arbitrary. The precursors of commutative and distributive properties that we described earlier do need to be refined, honed, extended, practiced, codified, and generalized, but they are already there as “natural” logic, the child’s natural habits of mind and the building blocks of higher mathematics. If children are to become competent at mathematics, including arithmetic, those habits of mind must take precedence over rules, formulas, and procedures that do not derive from logic that the child can grasp. In fact, children can grasp a lot more if the foundations for their learning are grounded in their logic, which gives them all the tools to understand, not just memorize, the algorithms for arithmetic with whole numbers and fractions. But we all see the dramatically disappointing results of “learning” rules without understanding: they are easy to mix up and result in procedures that don’t work. Organizing the arithmetic part of the elementary school mathematics curriculum around mathematical habits of mind would not shift the curriculum dramatically in content, except to give more attention to mental arithmetic than is usual. Paper and pencil methods are engineered to make the work easy, to reduce the cognitive load, the amount of thinking one needs to do, of calculation. Judiciously chosen mental arithmetic both exercises and depends on mathematical ways of thinking that the paper-and-pencil algorithms deliberately try to avoid, mathematical ways of thinking that are the backbone of the algebra that we want to prepare children to succeed at. What would shift is the order in which we acquire that content. Instead of being the preparatory step for computing, algorithms become the culmination of understanding how the computation works, another case of describing what we already know, and abbreviating that description.

References

An adaptation of this material is published as “An algebraic-habits-of-mind perspective on elementary school” in the NCTM Journal Teaching Children Mathematics, volume 16, number 9, pages 548-556. This work was supported in part by the National Science Foundation, grant numbers ESI-0099093, DRL-0733015, and DRL-0917958. The opinions expressed are those of the authors and not necessarily those of the Foundation.

Gopnik, A., Meltzoff, A., and P. Kuhl. The scientist in the crib: what early learning tells us about the mind. New York: HarperCollins. 2000.

Cuoco, A., Goldenberg, E. P., & J. Mark. “Habits of mind: an organizing principle for mathematics curriculum” J. Math. Behav. 15(4):375-402. December, 1996.

Goldenberg, E. Paul. “‘Habits of mind’ as an organizer for the curriculum” J. of Education 178(1):13-34, Boston U. 1996. (Also “‘Hábitos de pensamento’ …”Educação e Matemática, 47 March/April, & 48 May/June, 1998.)

Goldenberg, E. Paul & N. Shteingold. “Mathematical Habits of Mind.” In Lester, F., et al., eds. Teaching Mathematics Through Problem Solving: prekindergarten–Grade 6. Reston, VA: NCTM. 2003.

Goldenberg, E. Paul & N. Shteingold “The case of Think Math!” In Hirsch, Christian, ed., Perspectives on the design and development of school mathematics curricula. Reston, VA: NCTM. 2007.

Cuoco, A., Goldenberg, E. P., and J. Mark. “Organizing a curriculum around mathematical habits of mind.” Mathematics Teacher. (submitted)

8.0 8.1 Mark, J., Cuoco, A., and Goldenberg, E. P. “Developing mathematical habits of mind in the middle grades.” Mathematics Teaching in the Middle School. (submitted)

Education Development Center, Inc. (EDC). Think Math!, a comprehensive K-5 curriculum. Greenville, WI: School Specialty Math. 2008.

Feigenson, L., Carey, S., & Spelke, E. (2002). Infants’ discrimination of number vs. continuous extent. Cognitive Psychology, 44, 33–66.

Piaget, J. The child’s conception of number. London: Routledge and Kegan Paul. 1952.

Wirtz, R., Botel, M., Beberman, M., and W. W. Sawyer. 1964. Math Workshop. Encyclopaedia Britannica Press.

13.0 13.1 Sawyer, W. W. Vision in elementary mathematics. New York: Dover Publications. 2003 (1964).

Sfard, A. Thinking as Communicating. New York, NY: Cambridge University Press. 2008.