Although there are many kinds of symmetry, elementary school generally presents only reflective symmetry (or “mirror symmetry”). As a result, school materials tend to use the word symmetry as if it had only that one meaning. This article will focus on that one meaning, but illustrate others as well.

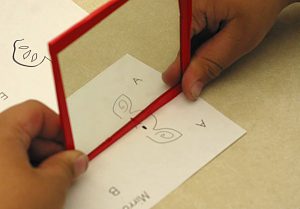
This photograph shows a simple picture with a mirror placed along the line of symmetry. The result that one sees — half of the original and the mirror image of that half — exactly matches the original figure. We say that the original figure is “symmetric” with respect to the mirror; it has reflective symmetry.

Reflective symmetry and “line of symmetry”

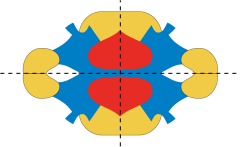
Mirror-symmetric objects have at least one line of symmetry, a line along which the figure can be folded into two precisely matching parts, parts that are mirror images of each other.

This figure has two lines of symmetry: the horizontal line of symmetry cuts the figure into a top and bottom that are mirror images of each other; the vertical line of symmetry cuts the figure into a left and right that are mirror images of each other. The star below has 5 lines of symmetry, five lines on which it can be folded so that both sides match perfectly.

A common misconception found even in many glossaries and texts: Not all lines that divide a figure into two congruent parts are lines of symmetry. For example, the diagonal of a (non-square) rectangle is not a line of symmetry.

When a mirror is placed along the diagonal of a rectangle, the result does not look the same as the original rectangle, so the diagonal is not a line of symmetry. This new shape — the combination of the triangular half of the original rectangle and its image in the mirror — is called a kite.

Mirror Play

Well before children begin any formal study of symmetry, playing with mirrors — perhaps on Pattern Block designs that they build — develops experience and intuition that can serve both their geometric thinking and their artistic ideas. The colorful design above has only vertical and horizontal lines of symmetry, but placing a mirror on it at another angle can create a beautiful new design.

The first figure below shows that the letter A has a vertical line of symmetry, but it’s rather “tame” play. More intrepid experiments give other interesting results.

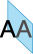
The same is true of the letter M.

Letters like B and D have a horizontal line of symmetry: their top and bottom parts match.

Some letters, for example, X, H, and O, have both vertical and horizontal lines of symmetry.

And some, like P, R, and N, have no lines of symmetry.

Rotational symmetry

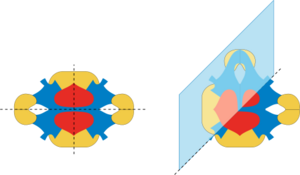
Another symmetry that children sometimes use in their Pattern Block designs is “Rotational Symmetry.” A figure has rotational symmetry if some rotation (other than a full 360° turn) produces the same figure. For example, these figures, when rotated just the right amount — 360°/3 for the “name” picture and 360°/5 for the star — look precisely as they did before rotation.

The letters, N, Z, and S also share that property.

Note that some figures, like the star and the colorful blob at the top of the page, but not the letters N, Z, or S, have both reflective and rotational symmetry.

The most symmetric shape

A circle has infinitely many lines of symmetry: any diameter lies on a line of symmetry through the center of the circle. Any rotation of any amount around the center of the circle also leaves the circle unchanged.

Books for children

• For young children, K-2:

Make a Bigger Puddle Make a Smaller Worm, by Marion Walter

• For older children, grade 2 and up:

The Mirror Puzzle Book, by Marion Walter

What’s in a word?

The “metry” in symmetry and geometry and metric refers to measurement.