Addition and Subtraction
Addition and subtraction of whole numbers can be developed together, systematically, starting with recognition of quantities and combinations of quantities, the 10-based structure of the language and symbols that represent whole numbers, convenient mental calculation methods that use the 10-based structure, and finally, formal algorithms for addition and subtraction.
The intent of this post is to help show part of the trajectory across grades.
Recognizing how many fingers
Fingers are a wonderful manipulative material. They’re fun, and they’re always at hand! But remember, the real point of any manipulative material is not to get the answers; it is to create ideas and images in the mind that allow the child to get those answers without the manipulative material.
Copy me (for the youngest children)
Using two hands, display some number of fingers. Ask children to make the same display with their hands. You can also extend this, when the children find that first game too easy, to “show the same number of fingers, but a different way! Children will be quite creative about making the correct combinations.
Make this number (for the youngest children)
Give some number from zero to 10. Ask children to show that number with their fingers. For example, if you say “three,” they might show

 or
or 

or any other two-hand combination that shows three fingers.

Kindergarten children may literally need some hand-holding to keep those little hands up and visible to the other children!
How many fingers? (first grade)
Using two hands, display some number of fingers. Ask children how many they see.
How many fingers don’t you see? (first and second grade)
Using two hands, display some number of fingers, showing the back of your hand like this.


Ask children how many they see. Then ask how many they don’t see (the ones that are folded down).
From the classroom
The Aha! moment: We have been working on ten frames in math, and trying to find all of the combinations to ten. It is a tedious process and we have been playing a game since the first day of school that Elementary Math Coordinator Russell Larson suggested. I have been holding up my fingers and saying, “how many do you see?” I just recently started asking, “how many don’t you see?” I was finally able to see it click! They finally understand what I mean when I say “6 and 4 are friends!” Patience and perseverance are everything! — Teacher, PISD, Texas
“Counting” what you don’t see
Much of elementary arithmetic is a system of shortcuts for knowing How Many or How Much without counting or measuring.
When everybody is present, we have 25 people in our class, but we only have 22 today. How many are absent?
We can’t count the people who are absent, because we don’t see them. Students can draw a picture so that they can see them, and use that to understand the problem. But we also want students to learn how to solve the problem without having to see the missing people.
There are 23 children in my first grade class, and there are 24 children in the other first grade class. How many in both?
Again, if all the children were standing in front of us, or if we drew a representation, we could count them. But the point of arithmetic is that we can know how many without counting.
Making mental pictures
One part of helping children to count what they don’t actually see is to help them to “see” inside their heads: to picture things, and to stretch the mental “space” that they can work in.
How many windows are in your room/apartment/house?
If you try to solve this problem, you probably take a mental walk around and count! There are many games that help young children increase their ability to make mental pictures. Here are three.
What did I see?
Hold up a picture for 15 seconds (or some suitable time, long enough for all to see, and not so long that your students get bored). It could be a large photo of some part of the room or school, or of a child in the class, or it could be a famous artwork.
Turn the picture face down and ask people to see the picture in their heads and recall as many details as they can.
Show how many
Hold your two hands up showing no fingers at all, then flash a number of fingers (like 7) for about one second — long enough to see, but not long enough to count, then go back to closed fists.
 then
then
 then
then
Then ask children to use their hands to show how many fingers you had up. (7, in this example)
Count in your head — two ways of playing
Fingers
- Start as in “Show how many,” showing a certain number of fingers just long enough to see, but not to count.
- Then ask “How many fingers did you see?”
Pictures
Make array-like pictures — you can draw them or use colorful stickers — with small numbers of objects (up to four or five) in each of up to three rows.
- Show the picture briefly, long enough to see, but not to count.
- Ask “How many dots (squares, stars, stickers…) did you see?”
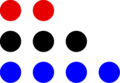 or
or  or
or  or
or
A game for young children
Excellent for kindergarten. First graders like this, too!
Gather children in on the floor so they can see and be close. In the center, arrange two dimes and five pennies in a row, like this, where all can see.

- How many pennies? (Some children will not know which are the pennies, or even that they are not all pennies, so you can clarify: “These copper-colored ones.”)
- How many dimes? (Again, you may need to clarify which are the dimes.)
- Oops! I forgot! How many pennies?
This is all so that the children have the chance to observe and count, and observe again before you cover some coins.

Now suddenly spring your hand playfully flat on the floor, covering two or three of the coins from either end.
- Can anyone tell how many coins I’ve hidden?! (Coins may be a new word for some. You could ask “how many pennies are hidden?” and “how many dimes are hidden?”)
Let them see, to check. Then do it again, perhaps at the other end.
- How many coins are hiding now?
Playfully change what you cover, sometimes covering one end, sometimes the other, sometimes the middle, sometimes nothing at all. Also playfully change your question, sometimes asking about coins, sometimes only about dimes dimes, or just pennies, or sometimes even worms. (You never cover any worms!)
Changing the question, and including silly questions (worms!) from time to time, encourages children to listen as well as look.
All about 10
Think Math! builds a strong foundation for addition and subtraction by systematically helping young students become fluent and automatic with
- making and breaking 10 — knowing “cold” the pairs of counting numbers that make ten: 1, 9; 2, 8; 3, 7; 4,6; and 5, 5
- adding and subtracting 10 starting at any number
- and using 10 as an approximation for nearby numbers (8 through 12) so that they can add or subtract those numbers as well.
Fluency with these skills aids mental computations and also helps students acquire other addition/subtraction facts and skills.
Making 10
Learning the 10-facts “cold” and then using them and extending them.
How many fingers don’t you see?
- Showing the back of your hand, display some number on your fingers, like this:


- Display a different number as soon as students respond.
Some fine points:
- While students are still “beginners” at this game, when you are showing numbers greater than 5, always show one complete hand and leftovers on the other hand.
In other words
 and not
and not

- Beginners often find it easier to know that two fingers are unseen when you show 8 than to realize that eight fingers are unseen when you show 2. Occasionally, alternate these, one after the other, to help them get the idea that 2 and 8 are a pair and that seeing either of them means that they do not see the other. Similarly with 3 and 7, and with 4 and 6.
- Even though you sometimes repeat pairs, alternating which number is shown (as was just described), use enough variety to keep the game fun.
How many dots don’t you see?
- Prepare a 4×6 card with two rows of five large dots. Large-size coding dots are nice for this. Use one color to minimize distraction (at least at first use).
- Cover that card in various ways for a lively-paced “How many don’t you see?”
 . . .
. . . . . .
. . . . . .
. . .
Pairs-to-ten: the “How many don’t you see?” game just using words
- You’re great with fingers! You’re great with dots! Can you do it just with words?!
- Say any number from 0 through 10. Students respond with the “pair” that makes 10.
For example, if you say 7, they say 3; if you say 0, they say 10.
- Keep the pace as lively as your class can go.
Fine points:
- If you make flash-cards with students names printed large enough for students to see, you can pick a student just by turning up a card. That way, they watch to see when it is their turn, and listen to hear the number you name. Say only the number, no other words.
- Occasionally, go back to a student who has already had a turn, so that students remain alert.
Extending “Pairs-to-10”
Here are several extensions for students who have become swift at “Pairs-to-ten”: Each of these extensions uses the skill that children have already developed, and also helps make that skill even more automatic.
How many dimes do you see?
This is one of the first extensions of the same idea.
The very same images that were used to teach the pairs to 10 can be recycled to work with higher numbers. Pretend that the fingers 
 or dots
or dots  are “dimes.”
are “dimes.”
- How many dimes do you see? How many dimes don’t you see? So far, this is exactly the same practice as before (and the same as if you had pretended the fingers or dots were apples, because you are merely counting the dimes, not thinking about how much they are worth).
- Then, for children who can count by 10s, you transition into helping them know how much three or four dimes are worth.
- Show three or four fingers, ask “how many dimes” as before.
- “Can anyone say how much money that is?” Sometimes children — even those who can do the arithmetic — don’t realize that a different question has been asked, so you may have to count your fingers as “ten cents, twenty cents, thirty cents…” to clarify what you mean.
- Show a different number of fingers (e.g., 7). First ask “how many dimes?” Children, having just seen (in the previous example) that you were asking about money may answer “70” instead of “7.” If so, acknowledge what question they answered correctly and that this tells you that they also know the answer to the question you asked, which was “how many dimes,” not “how much money.”
- Switch, irregularly, between “how many dimes” and “how much money” so that the children learn to notice that there are two different questions, and that they need to notice which one you asked.
- When they’re secure with that, add two more questions: “how many dimes don’t you see?” and “how much money don’t you see?”
Varying the questions also keeps the game lively. Children become as comfortable with 60 + 40 as they were with 6 + 4, but must stay alert to which of the four questions was asked. The fact that this is challenging can be a good giggle for child and adult alike.
- Finally, drop the visual cues. Announce that there are ten dimes total, and you’ll say how many dimes they see or how much money they see, and they’ll tell you what’s missing.
The parallel between these 100s facts and the 10s facts also builds (without any explanation) a strong sense of the orderliness of the way we write and name numbers.
Along with extending children’s skill to work with larger numbers, this further practices the same set of basic pairs, making them all the more automatic. It is important to include “ten dimes” and “zero dimes” along with the “100 cents” and “zero cents” and, at some point, to clarify when they pair 40 with 60 they are making pairs that add up to 100. Children quickly become adept at adding multiples of 10 that make 100.
Let’s make a thousand!
For many children at this point, it’s no challenge at all to take the next step up to 1000s (e.g., 300 + 700), and yet they feel proud and smart working with such big numbers! Using the same images, you can ask “How many hundreds do you see?” “How many hundreds don’t you see?” The game is the same, but now they are saying that “three hundreds” plus “seven hundreds” make a thousand (or, they may say, equally correctly, “ten hundreds.” You can also say that “three hundreds” is the number “three hundred,” but you probably won’t need even to say that. As children become experts at this, they also are building the foundation for being able to do much more challenging problems mentally.
Extending “Pairs-to-ten” to pairs to 20 or 30
Another way to extend is to make pairs to 20. Students still use the familiar pairings: 2 with 8; 6 with 4, and so on, but must also keep track of whether or not they need another 10. It helps if students are already comfortable adding 10 to any number (see below).
- It is often useful, and fun, to start with the concrete image of two sets of hands — twenty fingers — and some fingers down.
- Invite two children up to demonstrate. Explain to the class that you’ll whisper to the two demonstrators how many fingers to put down, and you’ll ask the class the usual questions: how many fingers are visible, or how many fingers are hiding.
- Whatever number you want the two children to “hide,” make sure that one child’s fingers are either all showing or all hidden so that the class is never trying to add numbers other than 10 and some number smaller than 10. (For example, if you want 14 fingers down, have the two demonstrators hide 10 and 4, not 7 and 7 or some other combination.)
- Vary your questions, making sure that you ask both about how many fingers the class sees and how many they don’t see, and that you ask both kinds of questions when children are hiding a number less than 10 and also when they are hiding a number greater than 10.
- Then, play the game without fingers, just saying a number from 0 through 20.
- You might invite one student to pick a number from 0 to 20. Then choose another student to give its pair. This allows you to differentiate instruction while all children participate in the same activity.
- When children are really good at this, try the same idea with pairs to make 30. The chances are that you won’t have to repeat the finger demonstration. Children might enjoy the challenge of a later extension to 40 or 50.
Extending “Pairs-to-ten” to use halves
- Start with whole numbers, as usual: 8, 2; 3, 7; 7, 3; 6, 4; and so on.
- After the class is comfortably doing the familiar thing, follow a 6 (to which they respond 4) with 6-and-a-half. Students are likely, at first, to respond incorrectly with “four-and-a-half,” but they are also likely to catch their own error, given a moment more to think about it, and answer “no! three-and-a-half!”
Second-graders, toward the end of the year, are likely to be able to learn to do this well. Even though this game deals only with small numbers (10 and under) and is using fractions, it is excellent preparation for adding and subtracting two-digit numbers mentally, and generally easier for children than the corresponding task in two digits (after hearing 60 and responding 40 to make 100, hearing 65 and responding 35).
Adding and subtracting 10
This section is being revised with new content. Please see Language and mathematics for the original contents of this section.
English, like most languages, names numbers in ways that make it easy (most of the time) to add or subtract ten “by rhyme.”[1] While there’s nothing obvious about “two, twelve, twenty-two,” the pattern after that just rhymes: twenty-two, thirty-two, forty-two, fifty-two… In general, in English, things are a bit messy before 20 but then clean up nicely. It’s not a “trick” for children to use this fact: the naming, after all, was a deliberate choice!
Chain 10 game
- One person names a number from 1 through 100 (e.g., 43) and the other person says the next (or previous) numbers, counting by 10, until they would go beyond 140 or below zero (e.g., 53, 63, 73, 83, 93, 103, 113, 123, 133 or, if the game is to go backwards, 33, 23, 13, 3).
↑ Some languages, like Japanese and Hungarian, are more regular; some, like French and Danish, are less regular.
Using 10 as an approximation
Under construction: using pairs that make 10 to ease computations with 8, 9, 11, 12, 80, 90, and so on
Fingers and facts from 11 through 20
Four-hand addition
Four hand addition or Leftovers, an activity for second grade
Call two children up and ask one to put up 6 fingers, the other to put up 8. This is what it might look like if they show the backs of their hands to the class.

 . . . .
. . . .

Then you pounce playfully on the two 5s, gather them to yourself, and ask the class, in rapid succession.
- How many do I have? (10)
- How many are left over? (4)
- How many altogether? (14)
Repeat with other pairs of children, using different pairs of numbers from 5 through 10 each time.
Children love it, and they’ll all want a turn. The ones who are demonstrating feel almost hugged when you pounce on the two fives and pull them slightly together. The whole class learns to see the “leftovers” — the excess over 5 — as a useful way of looking at numbers from 6 through 9.
A lot of important arithmetic learning and practice is involved. Just to put up eight fingers, a child must either count or know some fact that helps (5 + 3, 4 + 4, or perhaps even 10 – 8). To make sense out of the teacher’s pounce, the child practices the idea that two handfuls make 10. The child must be comfortable counting or adding up the leftovers (1 + 3), and must know the effect of adding 10 (the two handfuls) to another number smaller than 10 (the leftovers).
What if a child uses a combination that does not include a 5? For example, what if you ask for 6 and 8, and get

 . . . .
. . . .
You can still play. You might, for example, grab a 3 and a 4 and ask
- How many do I have? (7)
- How many left over? (7)
- Hmmm… So 7 and 7 is the same as 6 and 8! (By the way, how much is 7 + 7?)
But the purpose of the game is to practice ideas about making 10, and adding leftovers. So, also say,
From now on, we will show as many fingers as possible on one hand, and then whatever else is needed on the other hand.
Two-hand addition
Two-hand addition with Invisible fingers, an activity for second grade
Call one child up. Playfully ask the child to show 8 fingers on one hand. Of course, this is impossible, so, to the puzzled or amused expression, say…
- OK, well, show as many as you can!
- Perfect!
And now, playfully, to the class…
- Excellent! She’s put up eight figures! Do you see them all? No?! Well, how many do you see? (5)
- Right! The other fingers are invisible! How many invisible fingers does Shira have up? (3)
- OK, Shira. On your other hand, please put up 7 fingers!
Children often “get” the silly game this quickly, but if necessary help again by saying “Show as many as you can.”
- So, class, Shira has put up 8 fingers on this hand, and 7 fingers on that hand.
- Let’s see… How many invisible fingers on this hand? (3)
- And how many invisible fingers on that hand? (2)
- Hmm… That’s a lot of invisible fingers!
By not asking yet how many invisible fingers in total, you “extend” the time that children are keeping this in their heads.
- How many visible fingers does Shira have up? (10)
- And how many invisible ones? (5)
- So how many all together? (15)
- Yes! Fifteen fingers: eight here and seven there!
Many children will enjoy getting a turn, and that gives the class plenty of practice keeping mental track of invisible fingers.
