Informally: When you multiply an integer (a “whole” number, positive, negative or zero) times itself, the resulting product is called a square number, or a perfect square or simply “a square.” So, 0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, and so on, are all square numbers.
More formally: A square number is a number of the form n × n or n2 where n is any integer.
Mathematical background
Objects arranged in a square array
The name “square number” comes from the fact that these particular numbers of objects can be arranged to fill a perfect square.
Children can experiment with pennies (or square tiles) to see what numbers of them can be arranged in a perfectly square array.
Four pennies can: 
Nine pennies can: 
And sixteen pennies can, too: 
But seven pennies or twelve pennies cannot be arranged that way. Numbers (of objects) that can be arranged into a square array are called “square numbers.
Square arrays must be full if we are to count the number as a square number. Here, 12 pennies are arranged in a square, but not a full square array, so 12 is not a square number.

The number 12 is not a square number.
Children may enjoy exploring what numbers of pennies can be arranged into an open square like this. They are not called “square numbers” but do follow an interesting pattern.
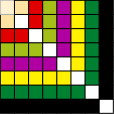
Squares made of square tiles are also fun to make. The number of square tiles that fit into a square array is a “square number.”

Here are two boards, 3 × 3 and 5 × 5. How many red tiles in each? Black? Yellow?
Are any of those square numbers?
What if you tile a 4 × 4 or 6 × 6 board the same way?
Can you predict the number of tiles in a 7 × 7 or 10 × 10 board?
Square numbers in the multiplication table
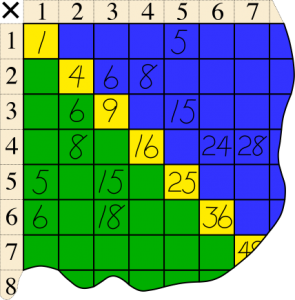
Square numbers appear along the diagonal of a standard multiplication table.
Connections with triangular numbers
If you count the green triangles in each of these designs, the sequence of numbers you see is: 1, 3, 6, 10, 15, 21, …, a sequence called (appropriately enough) the triangular numbers.
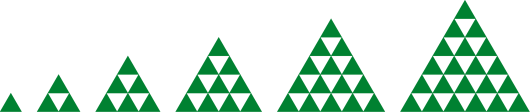 If you count the white triangles that are in the “spaces” between the green ones, the sequence of numbers starts with 0 (because the first design has no gaps) and then continues: 1, 3, 6, 10, 15, …, again triangular numbers!
If you count the white triangles that are in the “spaces” between the green ones, the sequence of numbers starts with 0 (because the first design has no gaps) and then continues: 1, 3, 6, 10, 15, …, again triangular numbers!
Remarkably, if you count all the tiny triangles in each design—both green and white—the numbers are square numbers!
A connection between square and triangular numbers, seen another way
Build a stair-step arrangement of Cuisenaire rods, say W, R, G. Then build the very next stair-step: W, R, G, P. 
Each is “triangular” (if we ignore the stepwise edge). Put the two consecutive triangles together, and they make a square:  . This square is the same size as 16 white rods arranged in a square. The number 16 is a square number, “4 squared,” the square of the length of the longest rod (as measured with white rods).
. This square is the same size as 16 white rods arranged in a square. The number 16 is a square number, “4 squared,” the square of the length of the longest rod (as measured with white rods).
Here’s another example:  . When placed together, these make a square
. When placed together, these make a square 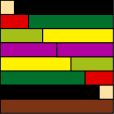 whose area is 64, again the square of the length (in white rods) of the longest rod. (The brown rod is 8 white rods long, and 64 is 8 times 8, or “8 squared.”)
whose area is 64, again the square of the length (in white rods) of the longest rod. (The brown rod is 8 white rods long, and 64 is 8 times 8, or “8 squared.”)
Stair steps from square numbers

Stair steps that go up and then back down again, like this, also contain a square number of tiles. When the tiles are checkerboarded, as they are here, an addition sentence that describes the number of red tiles (10), the number of black tiles (6), and the total number of tiles (16) shows, again, the connection between triangular numbers and square numbers: 10 + 6 = 16.
Inviting children in grade 2 (or even 1) to build stair-step patterns and write number sentences that describe these patterns is a nice way to give them practice with descriptive number sentences and also becoming “friends” with square numbers.
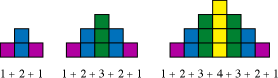
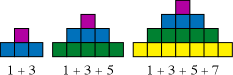
Here are two examples. Color is used here to help you see what is being described. Children enjoy color, but don’t need it, and can often see creative ways of describing stair-step patterns that they have built with single-color tiles. Or they might color on 1″ graph paper to record their stair-step pattern, and show how they translated it into a number sentence.

A diamond-shape made from pennies can also be described by the 1 + 2 + 3 + 4 + 5 + 4 + 3 + 2 + 1 = 25 number sentence.
From one square number to the next: two images with Cuisenaire rods
(1) Start with W. Add two consecutive rods, W+R; then another two, R+G; then G+P; then….
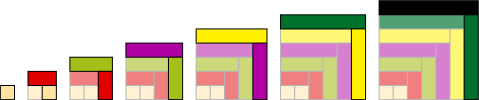
| 1; | add 1+2; | add 2+3; | add 3+4; | add 4+5; | add 5+6; | add 6+7 |
(2) Start with W. For each new square, add two rods that match the sides of the previous square, and a new W to fill the corner.